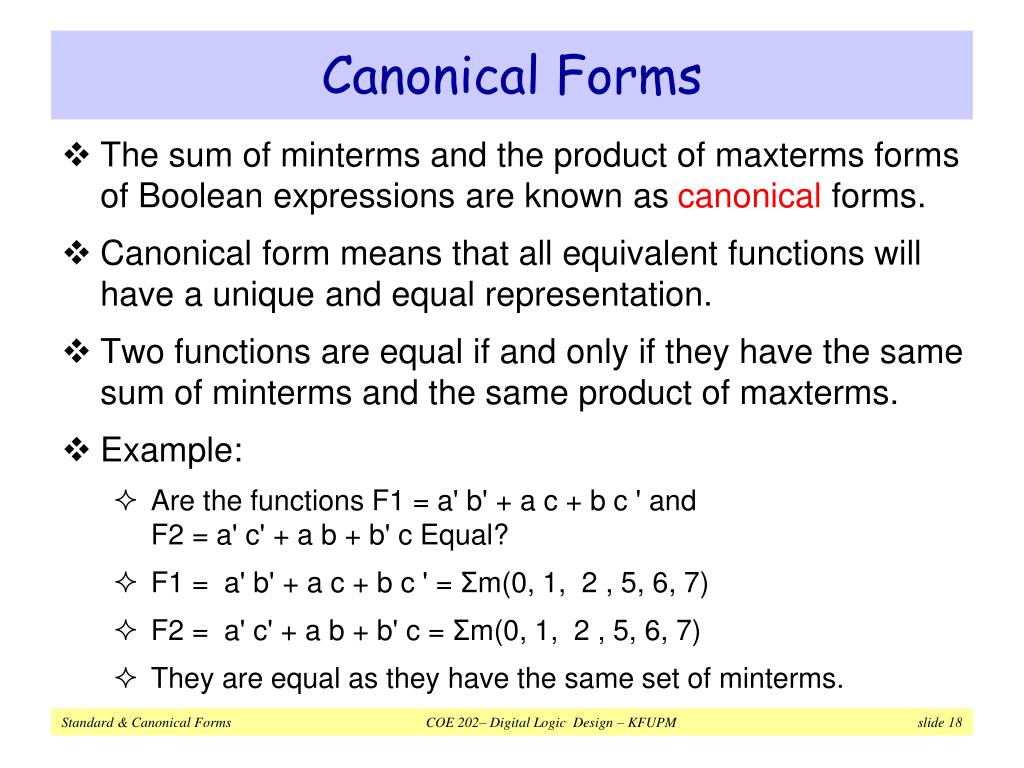
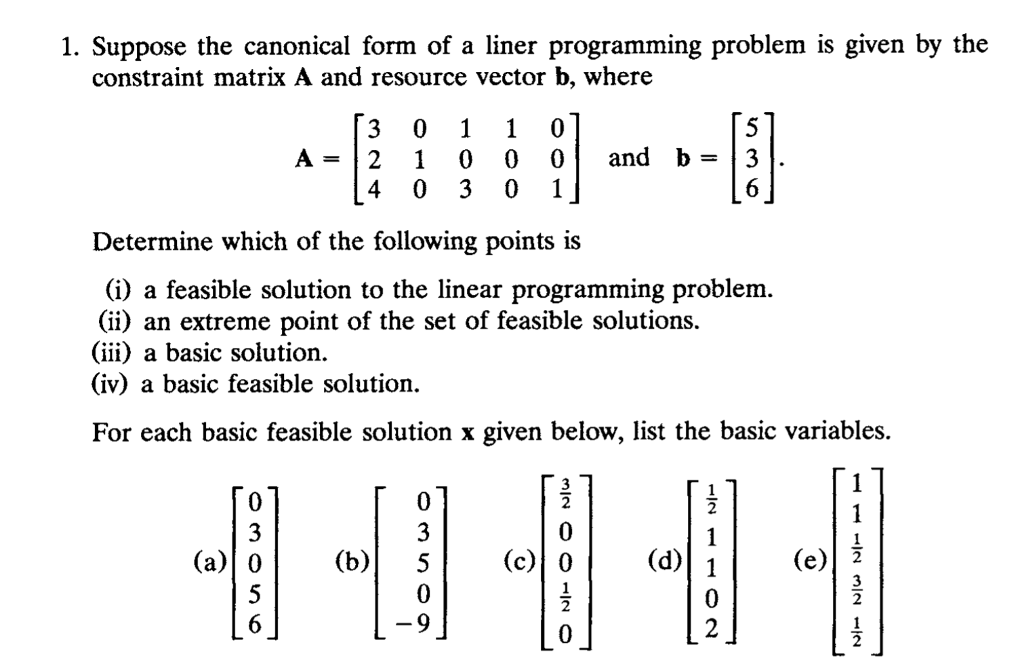
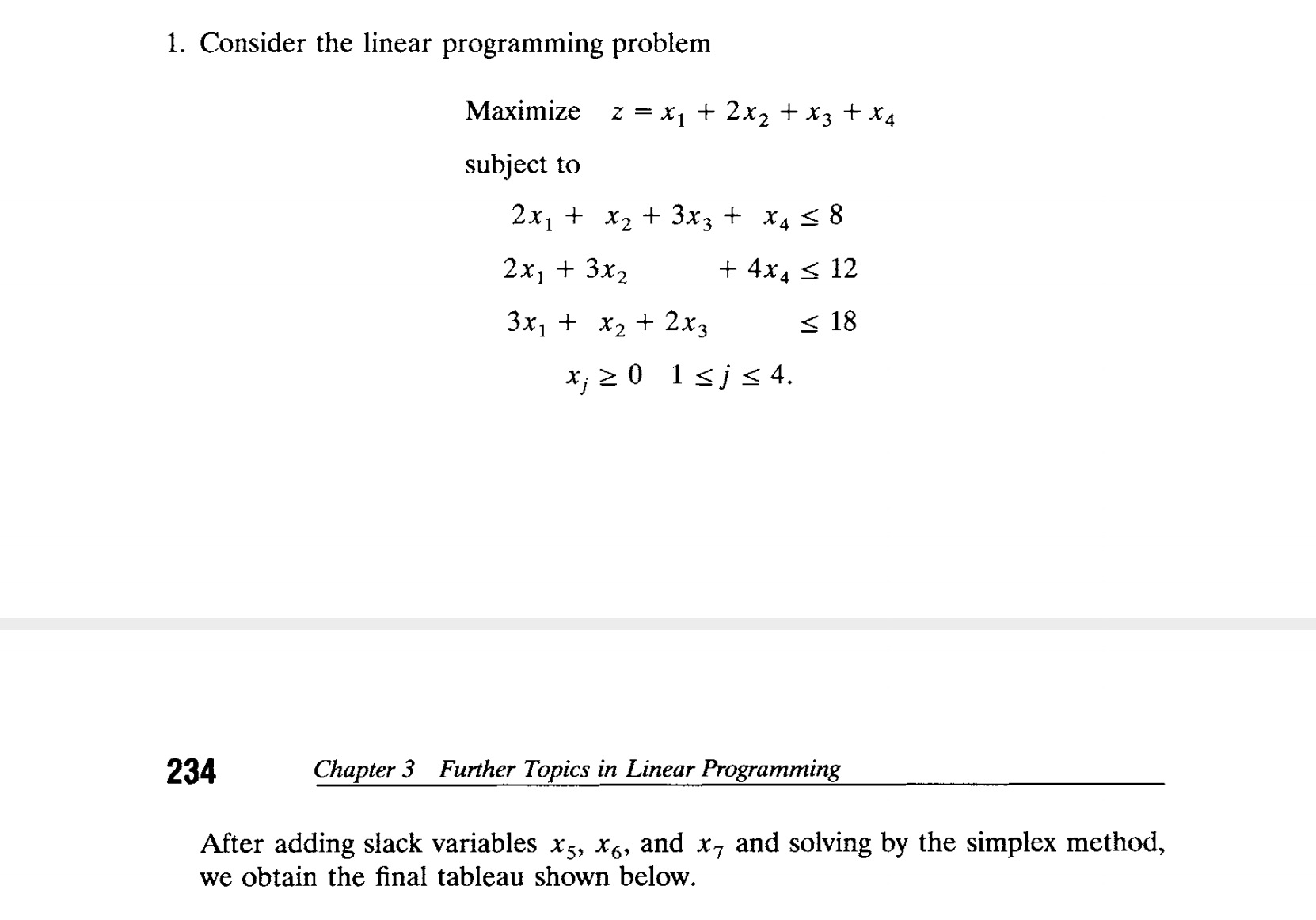
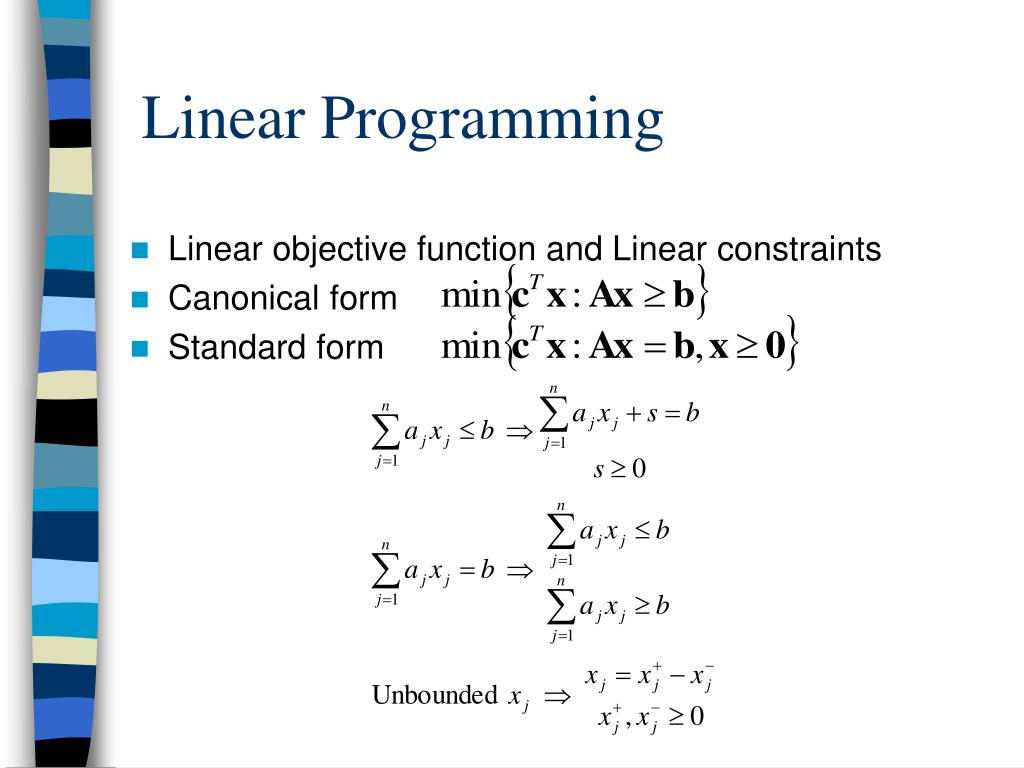
Canonical Form Linear Programming
Canonical Form Linear Programming - One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. For example x = (x1, x2, x3) and. A linear program is said to be in canonical form if it has the following format: A linear program in standard. To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$.
A linear program in standard. A linear program is said to be in canonical form if it has the following format: For example x = (x1, x2, x3) and. In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms. One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$. A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s.
In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. A linear program in standard. A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. For example x = (x1, x2, x3) and. A linear program is said to be in canonical form if it has the following format: One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$.
PPT Standard & Canonical Forms PowerPoint Presentation, free download
To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms. For example x = (x1, x2, x3) and. A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. One canonical form.
Canonical Form (Hindi) YouTube
In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. A linear program is said to be in canonical form if it has the following format: To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms. Maximize $c^tx$ subject to.
PPT Standard & Canonical Forms PowerPoint Presentation, free download
For example x = (x1, x2, x3) and. A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. A linear program is said to be.
Theory of LP Canonical Form Linear Programming problem in Canonical
A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. A linear program is said to be in canonical form if it has the following format: Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and.
Solved 1. Suppose the canonical form of a liner programming
A linear program in standard. For example x = (x1, x2, x3) and. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$. A linear program is said to be in canonical form if it has the following format: A linear program in canonical form can be replaced by a linear program in standard form by.
Canonical Form of a LPP Canonical Form of a Linear Programming
Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$. In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. A linear program is said to be in canonical form if it has the following format: To describe properties of and algorithms for linear.
1. Consider the linear programming problem Maximize
A linear program in standard. For example x = (x1, x2, x3) and. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$. One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms.
OR Lecture 28 on Canonical and Standard Form of Linear Programming
For example x = (x1, x2, x3) and. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$. A linear program in standard. One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms.
PPT Representations for Signals/Images PowerPoint
A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. A linear program is said to be in canonical form if it has the following format: To describe properties of and algorithms for linear programs, it is convenient to.
PPT Linear Programming and Approximation PowerPoint Presentation
In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. A linear program is said to be in canonical form if it has the following format: Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$. A linear program in canonical form can be.
For Example X = (X1, X2, X3) And.
One canonical form is to transfer a coefficient submatrix into im with gaussian elimination. A linear program in standard. In canonical form, the objective function is always to be maximized, every constraint is a ≤ constraint, and all variables are implicitly. To describe properties of and algorithms for linear programs, it is convenient to express them in canonical forms.
A Linear Program Is Said To Be In Canonical Form If It Has The Following Format:
A linear program in canonical form can be replaced by a linear program in standard form by just replacing ax ≤b by ax + is = b, s ≥0 where s. Maximize $c^tx$ subject to $ax ≤ b$, $x ≥ 0$ where $c$ and $x$.