Elipse Standard Form
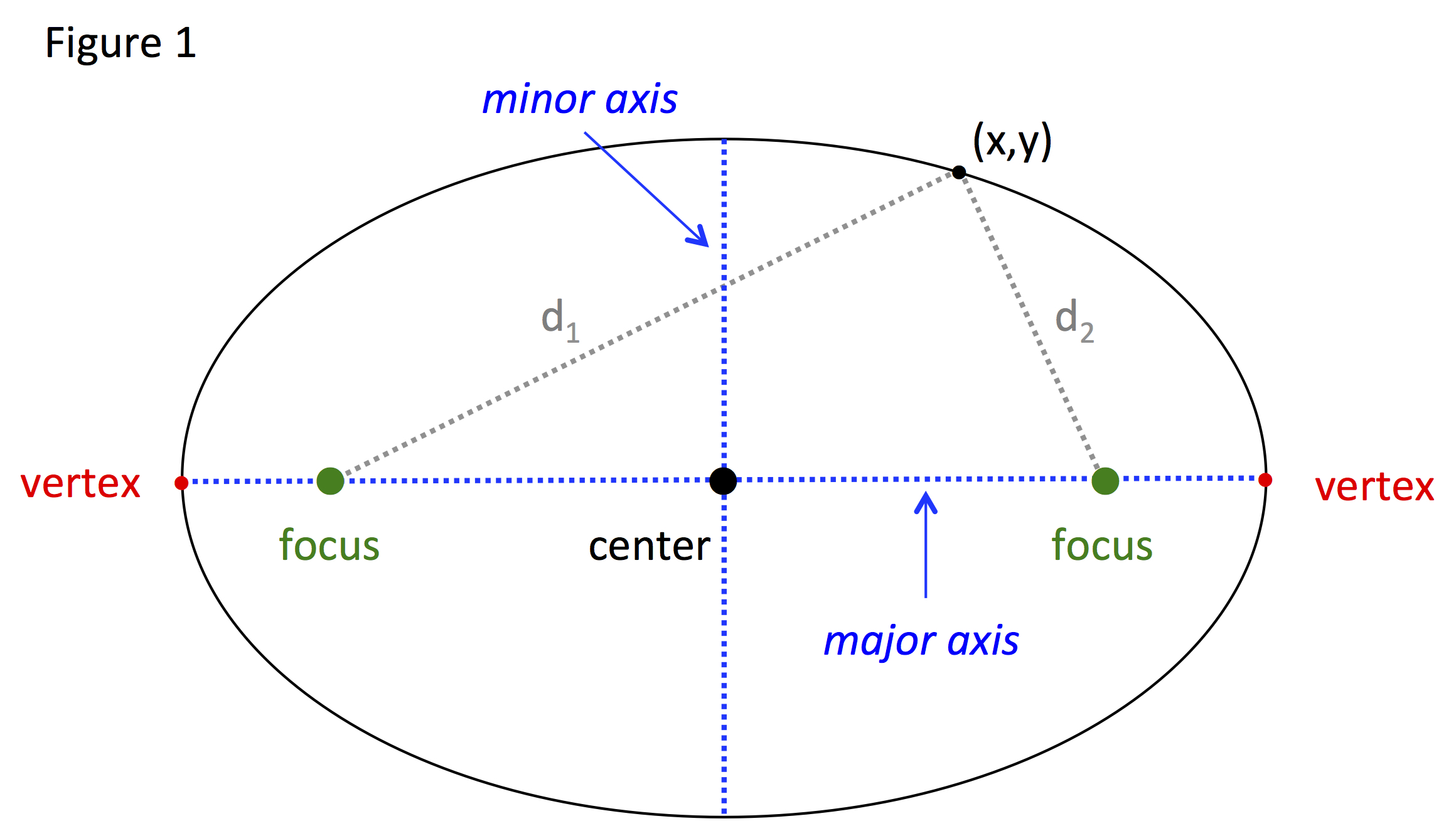
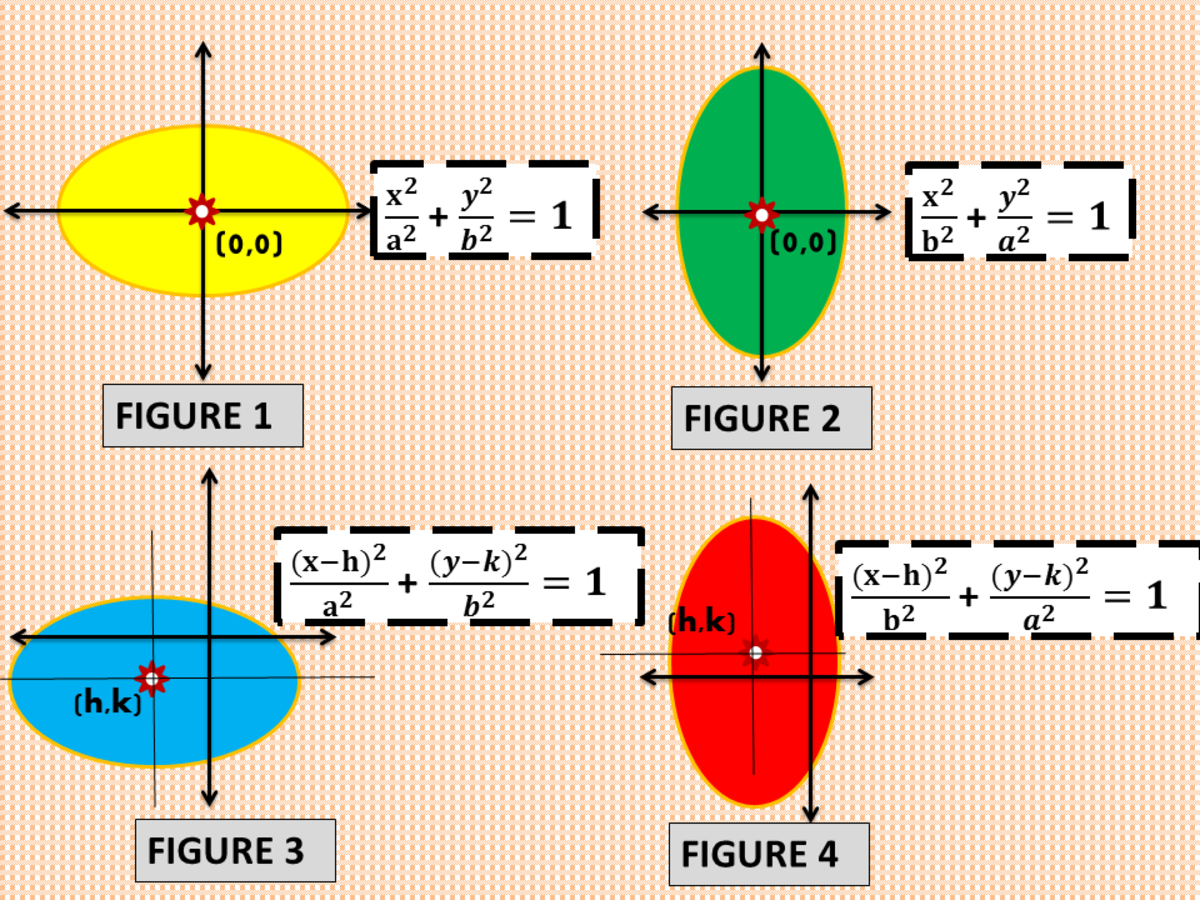
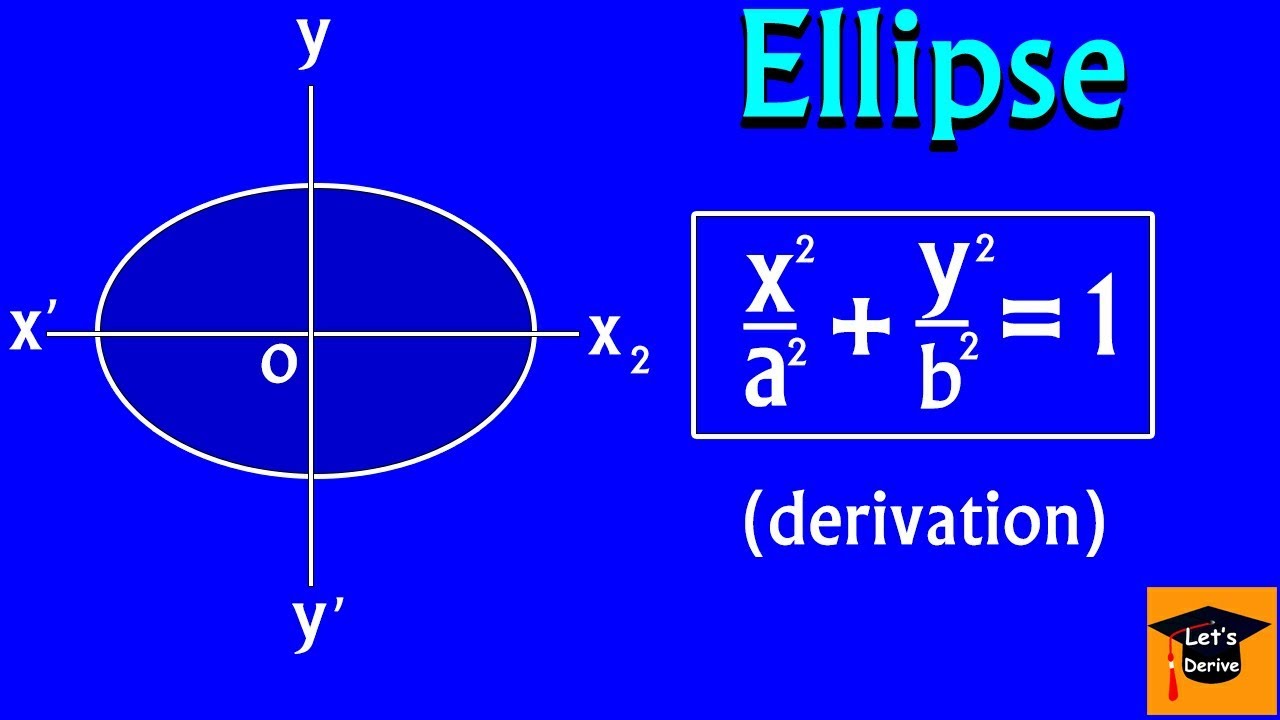
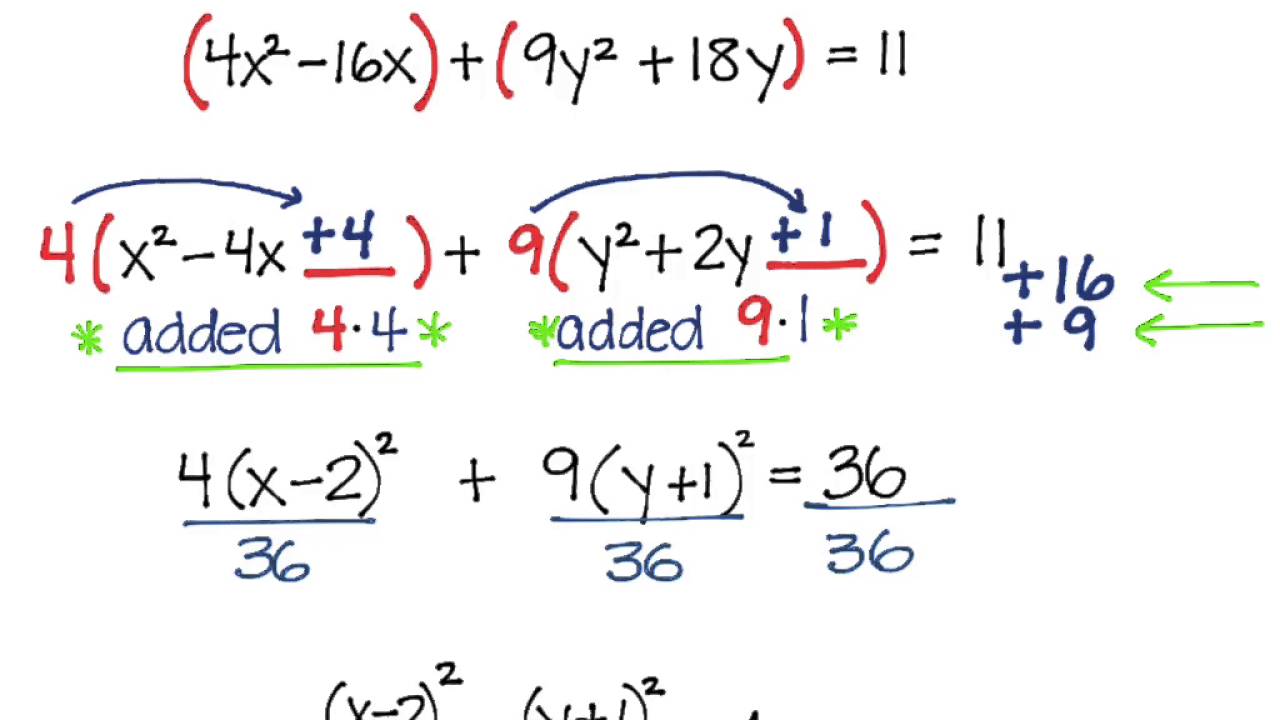Elipse Standard Form - The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. The standard form of an ellipse in cartesian coordinates assumes that the origin is the center of the ellipse,. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. This form can be converted to the.
First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. This form can be converted to the. The standard form of an ellipse in cartesian coordinates assumes that the origin is the center of the ellipse,. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =.
The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. This form can be converted to the. The standard form of an ellipse in cartesian coordinates assumes that the origin is the center of the ellipse,. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =.
Ellipse Standard Equation
The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. First we will learn to derive the equations of ellipses, and then we will learn how to write the.
Ellipse Formula General Form
The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} =.
Determine the general form of equation of an elipse whose standard form
This form can be converted to the. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. The standard form of an ellipse in cartesian coordinates assumes that the.
Ellipse Equation, Properties, Examples Ellipse Formula
The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. This form can be converted to the. The standard form of an ellipse in cartesian coordinates assumes that the origin is the center of the ellipse,. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}}.
Conics Standard Form of an Ellipse Expii
First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The standard form of an ellipse in cartesian coordinates assumes that the origin is the center of the ellipse,. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2.
92 Ellipse into standard form from equation YouTube
The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. This form can be converted to the. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. First we will learn to derive the equations of ellipses, and then.
ellipse general form to standard form YouTube
This form can be converted to the. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. The standard form is \frac {x^ {2}} {3^ {2}}.
How to Graph an Ellipse Given an Equation Owlcation
This form can be converted to the. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. The standard form of an ellipse.
Writing Equations of Ellipses In Standard Form and Graphing Ellipses
This form can be converted to the. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The standard form is \frac {x^ {2}} {3^ {2}}.
Equation Of Ellipse derivation YouTube
First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. The vertex form is \frac {x^ {2}} {9} + \frac {y^ {2}} {4} = 1 9x2 + 4y2 =. This form can be converted to the. The standard form of an ellipse in cartesian coordinates.
The Vertex Form Is \Frac {X^ {2}} {9} + \Frac {Y^ {2}} {4} = 1 9X2 + 4Y2 =.
The standard form is \frac {x^ {2}} {3^ {2}} + \frac {y^ {2}} {2^ {2}} = 1 32x2 + 22y2 = 1. The standard form of an ellipse in cartesian coordinates assumes that the origin is the center of the ellipse,. First we will learn to derive the equations of ellipses, and then we will learn how to write the equations of ellipses in standard form. This form can be converted to the.